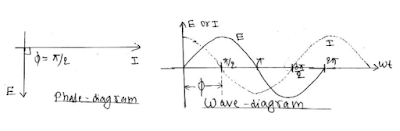
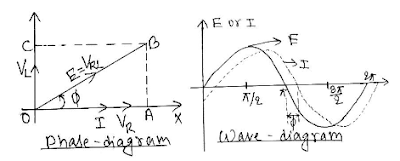
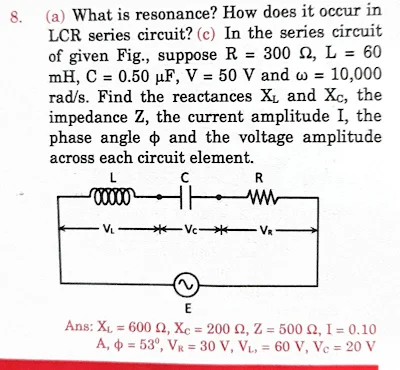
Monday, August 28, 2023
AC
Subscribe to:
Posts (Atom)
Featured Post
Elasticity
Elasticity a Elasticity Elasticity is the property of the body by virtue of which a deformed body regains its original shape, size and posi...
-
Cell division project Mitosis Cell Division Meiosis Cell Division Differences a VISUALIZATION OF CELL DIVISIO...